
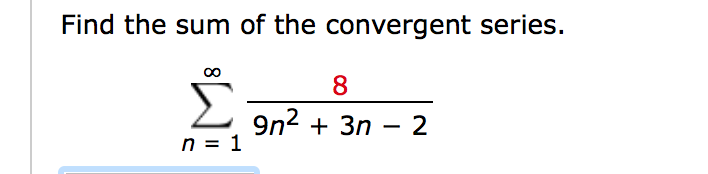
In higher math, series plays an important role in limits in Calculus.In business, the idea geometric sequence and series is used to represent and predict data.Understanding the concepts behind geometric series is a good foundation in learning about investment growth and loss.FORMULA IN GETTING THE SUM OF GEOMETRIC SERIES.CONVERGENT GEOMETRIC SERIES – A series that converges has a finite limit, that is a number that is approached.GEOMETRIC SERIES – It is the sum of the terms of a geometric sequence.To solve problems involving geometric series such as investment and compound interest.To differentiate convergent and divergent series.To define geometric series and related concepts.Word Problems Involving Geometric Series.The sum of an infinite GP converges when the absolute value of the common ratio of GP is less than 1. When Does the Sum of an Infinite GP Converge? We cannot find the sum of infinite terms of such GP. The sum of finite (n) terms of such GP = a + a + a +. If r = 1, then the GP is of form a, a, a. What is the Geometric Progression Sum Formula with r = 1? In case, |r| ≥ 1 for an infinite GP, then it diverges and hence we cannot find its sum. If GP is infinite, then we can find its sum only when |r| < 1 and we use the formula S ∞ = a/(1-r) in this case. What is the Sum of Geometric Progression Formula for Infinite Terms? If r = 1, then the sum of n terms is S n = n These two formulas would work when r ≠ 1. The sum of n terms in GP with 'a' to be its first term and 'r' to be its common ratio can be found using one of the formulas: The sum of an infinite GP diverges when the absolute value of the common ratio of GP is either equal to 1 or greater than 1. When Does the Sum of an Infinite GP Diverge? The GP sum formula used to find the sum of n terms in GP is, S n = a(r n - 1) / (r - 1), r ≠ 1 where: The sum of GP (of infinite terms) is: S ∞ = does not exist, when |r| ≥ 1.įAQs on GP Sum What is the Formula of Finding GP Sum for Finite Terms?.The sum of GP (of infinite terms) is: S ∞ = a/(1-r), when |r| The sum of GP (of n terms) is: S n = na, when r = 1.The sum of GP (of n terms) is: S n = a(r n - 1) / (r - 1) S n = a(1 - r n) / (1 - r), if r ≠ 1.is an infinite geometric series with a = 1 and r = 1/2. Taking 's 2' as common factor, the sum of areas is, s 2 ( 1 + 1/2 + 1/4 +.
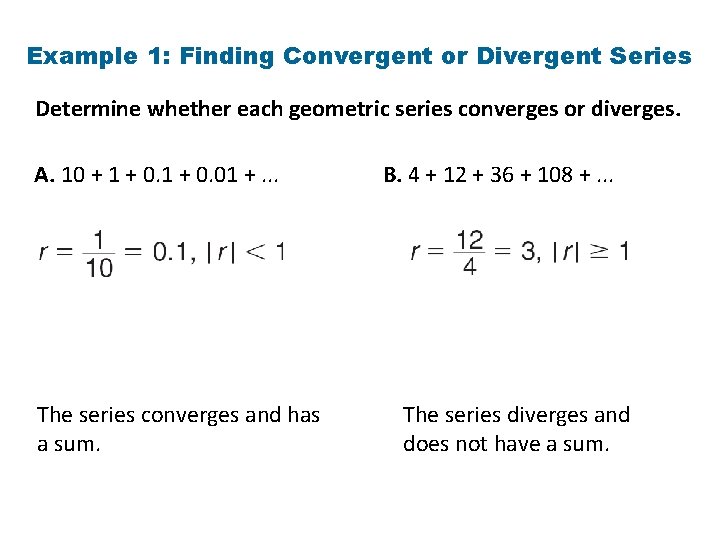
The areas of squares thus formed are, s, s 2/2, s 2/4, s 2/8. If a side of the first square is "s" units, determine the sum of areas of all the squares so formed? A third square is drawn inside the second square in the same way and this process is continued indefinitely. If |r| ≥ 1, then the geometric series diverges and it cannot have a sum.Įxample: A square is drawn by joining the midpoints of the sides of the original square.If |r|

Note that for any infinite geometric series, This is the formula for the sum of infinite GP. Let us assume the sum of all these infinite number of terms be S ∞. (up to an infinite number of terms) such that the absolute value of its common ratio is less than 1. But what if we have to find the sum of an infinite GP? Let us consider a GP a, ar, ar 2. The above formula gives the sum of a finite GP. We have got the same answer using the GP sum formula also. Here, the first term is, a = 2, the common ratio is, r = 2, and the number of terms is, n = 6 (as we want the sum of the amounts after 6 weeks). Clearly, this is a geometric progression as 4/2 = 8/4 = 16/2 =. The amounts saved by Clara in the order of weeks are, 2, 4, 8, 16. Now, let us work on the example (from the last section) using the sum of n terms of GP formula. What happens when r = 1? Then the GP is of form a, a, a. Subtracting equation (1) from equation (2): Let S be the sum of the geometric progression of n terms.
Then the first 'n' terms of GP are of the form a, ar, ar 2. Consider the sum of the first n terms of a geometric progression (GP) with first term a and common ratio r.
SUM OF GEOMETRIC SEQUENCE CONVERGE HOW TO
Let's discuss how to calculate the sum of n terms of GP.


 0 kommentar(er)
0 kommentar(er)
